Are you a B.Tech student at AKTU studying fluid mechanics? If that’s the case, you’ve come to the correct spot. We’ve compiled a list of quick questions that you should be aware of. Let’s get started.
Dudes 🤔.. You want more useful details regarding this subject. Please keep in mind this as well. Important Questions For Fluid Mechanics: *Unit-01 *Unit-02 *Unit-03 *Unit-04 *Unit-05 *Short-Q/Ans *Question-Paper with solution 21-22
Unit – 1 (Fluid and Continuum)
Q1. What is an ideal fluid ?
Ans. The absence of viscosity, surface tension, and compressibility characterize a perfect fluid.
Q2. Define control volume.
Ans. The control volume approach, in which a specific volume with a set boundary shape is chosen in space along the fluid flow path, is typically used to apply fundamental fluid flow concepts. Control volume refers to this specific volume.
Q3. Define viscosity.
Ans. The characteristic of a fluid that influences how resistant it is to shearing stresses is known as viscosity. It serves as a gauge for the internal fluid friction that produces flow resistance.
Q4. What is kinematic viscosity?
Ans. The dynamic viscosity to fluid density ratio is known as kinematic viscosity. It is denoted by v.
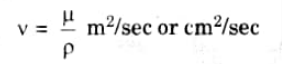
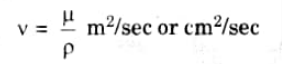
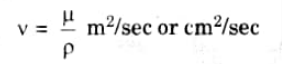
Q5. State the Newton’s law of viscosity.
Ans. According to the law, the rate of shear strain is directly proportional to the shear stress on a fluid element layer. The coefficient of viscosity is the name for the proportionality constant. Mathematically,



Q6. What are Newtonian fluids ?
Ans. Fluids that adhere to Newton’s law of viscosity are referred to as Newtonian fluids. The viscosity of these fluids does not alter with the rate of deformation.
Q7. Define non-Newtonian fluids.
Ans. Non-Newtonian fluids are defined as those that defy Newton’s viscosity law.
Q8. Define surface tension.
Ans. Surface tension is the tensile force that acts on a liquid’s surface when it comes into contact with a gas or on the surface between two immiscible liquids, causing the surface to behave like a membrane under tension.
Q9. State the hydrostatic law.
Ans. The law indicates that the weight density of the fluid equals the rate of increase of pressure in a vertical downward direction. Thus,
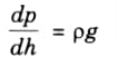
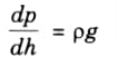
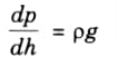
where, h = Distance of fluid element from free surface
vertical downward direction
ρ = Density of fluid, and
g = Acceleration due to gravity.
Q10. State the Pascal’s law.
Ans. According to Pascal’s law, pressure is equally intense in all directions at any point in a liquid that is at rest.
Q11. Define gauge pressure and absolute pressure.
Ans. Gauge Pressure: A gauge pressure is a pressure that is measured using a pressure measuring device with the atmospheric pressure serving as the reference point.
Absolute Pressure: Absolute pressure is any pressure that is measured above the zero-point pressure.
Q12. Define piezometer.
Ans. The most basic type of manometer that can be used to measure liquid pressure at a reasonable level is a piezometer.
Q13. What is centre of pressure ?
Ans. The place at which all of the surface’s pressure is applied is known as the centre of pressure.
Q14. What do you understand by buoyant force ?
Ans. The buoyant force is the hydrostatic lift caused by the body’s net vertical hydrostatic pressure force when it is either fully or partially submerged in a fluid.
Q15. What do you mean by centre of buoyancy?
Ans. The centre of buoyancy is the location where the force of buoyancy acts on the body.
Q16. Define specifie volume.
Ans. The volume of a fluid occupied by a unit mass or the volume of a fluid per unit mass is referred to as the specific volume of a fluid. Mathematically, it is expressed as,



Thus specific volume is the reciprocal of mass density. It is expressed as m3/kg. It is commonly applied to gases.
Q17. What is metacentric height ? How is it determined ?
Ans. Metacentric Height: Metacentric height is the distance between a body’s centre of gravity and the metacentre.
It can be calculated by,
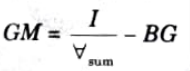
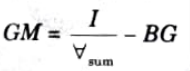
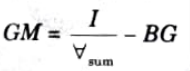
Metacentre : When the body is given a slight angular displacement, the line of action of the buoyancy force will intersect the normal axis of the body at the metacentre.
Q18. Define weight density.
Ans. It can be characterised as the mass per unit volume at standard pressure and temperature. It also goes by the name “specific weight.”
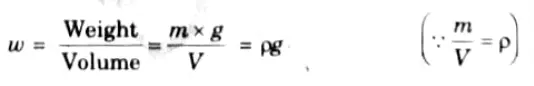
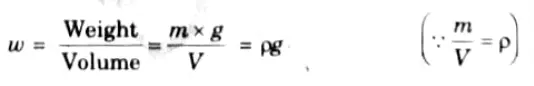
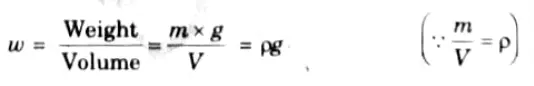
Q19. Define perfect gas.
Ans. A perfect gas is one that precisely abides by the gas low under all conditions of pressure and temperature.
Q20. What do you understand by stable equilibrium ?Ans. When a body is in an equilibrium state, even if it is slightly moved, it tends to return to its original position
Unit – 2 (Types of Fluid and Continuity Equation)
Q1. Classify the fluid flow.
Ans. Fluid flow may be classified as follows:
- 1. Steady and unsteady flows.
- 2. Uniform and non-uniform flows.
- 3. One, two and three dimensional flows.
- 4. Rotational and irrotational flows.
- 5. Laminar and turbulent flows.
- 6. Compressible and incompressible flows.
Q2. Differentiate between steady and unsteady flow.
Ans. Steady Flow : Stable flow is a form of flow in which the fluid parameters at a given site, such as velocity, pressure, density, etc., do not fluctuate over time. Mathematically,
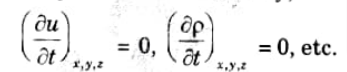
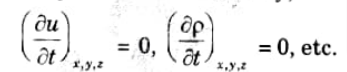
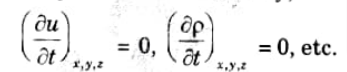
Unsteady Flow : Unsteady flow is the kind of flow when the speed, pressure, or density at a given place varies over time. Mathematically,
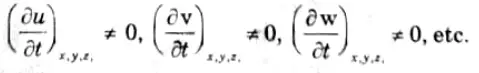
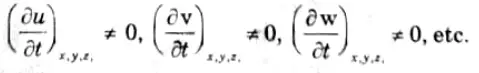
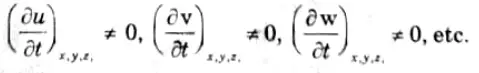
Q3. Explain uniform and non-uniform flow.
Ans. Uniform Flow: The type of flow, in which the velocity at any given time does not change with respect to space, is called uniform flow.
Mathematically,



Non-Uniform Flow: Non-uniform flow is that type of flow in which the velocity at any given time changes with respect to space.
Q4. What is laminar and turbulent flow ?
Ans. Laminar Flow: Laminar flow is a flow in which individual particle pathways do not intersect one another and travel along clearly defined paths.
Turbulent Flow: The flow in which fluid particles move zigzag-style is known as a turbulent flow.
Q5. Distinguish between rotational and irrotational flow?
Ans. Rotational Flow : If fluid particles travelling in the direction of the flow rotate about their mass centres, the flow is said to be rotational.
Irrotational Flow : If the fluid particles do not rotate around their mass centres while travelling in the direction of the flow, the flow is said to be irrotational.
Q6. Discuss compressible and incompressible flow.
Ans. Compressible Flow : The type of flow known as compressible flow occurs when the fluid’s density varies from one location to another.
Incompressible Flow : In the field of fluid mechanics, an incompressible flow is one in which the material density within a fluid parcel remains constant.
Q7. Define sub-sonie, sonic and supersonic flow?
Ans. 1. When Mach number is less than 1 (M< 1), flow is called subsonic flow.
2. When Mach number is equal to 1 (M = ), flow is called sonic flow.
3. When Mach number is greater than 1 (M > 1), flow is called supersonic flow.
Q8. Define sub-critical, critical and supercritical flow.
Ans. 1. When Froude number is less than one (Fe < ), the flow is subcritical flow.
2. When Froude number is equal to one (Fe = 1), the flow is critical flow.
3. When Froude number is greater than one (Fe > 1), the flow is supercritical flow.
Q9. Define streamline.
Ans. A streamline is a hypothetical line within the flow where the tangent at any point on the line represents the velocity there.
Q10. What is stream tube ?
Ans. A fluid mass enclosed by a collection of streamlines is called a stream tube. Current filament refers to a stream tube’s contents.
Q11. What do you understand by circulation ?
Ans. The line integral of the tangential velocity about a closed path is what is known as circulation (contour). Integration can be used to obtain the circle’s path around regular curves.
Q12. Write down the definition of stream function ?
Ans. A stream function is a function of space and time that produces a velocity component that is at right angles to any direction when its partial derivative is applied to it. It is denoted by ψ
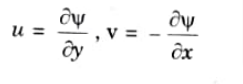
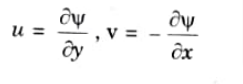
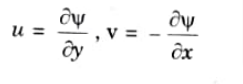
Mathematically,



Q4. What is laminar and turbulent flow ?
Ans. Laminar Flow: Laminar flow is a flow in which individual particle pathways do not intersect one another and travel along clearly defined paths.
Turbulent Flow: The flow in which fluid particles move zigzag-style is known as a turbulent flow.
Q5. Distinguish between rotational and irrotational flow?
Ans. Rotational Flow : If fluid particles travelling in the direction of the flow rotate about their mass centres, the flow is said to be rotational.
Irrotational Flow : If the fluid particles do not rotate around their mass centres while travelling in the direction of the flow, the flow is said to be irrotational.
Q6. Discuss compressible and incompressible flow.
Ans. Compressible Flow : The type of flow known as compressible flow occurs when the fluid’s density varies from one location to another.
Incompressible Flow : In the field of fluid mechanics, an incompressible flow is one in which the material density within a fluid parcel remains constant.
Q7. Define sub-sonie, sonic and supersonic flow?
Ans. 1. When Mach number is less than 1 (M< 1), flow is called subsonic flow.
2. When Mach number is equal to 1 (M = ), flow is called sonic flow.
3. When Mach number is greater than 1 (M > 1), flow is called supersonic flow.
Q8. Define sub-critical, critical and supercritical flow.
Ans. 1. When Froude number is less than one (Fe < ), the flow is subcritical flow.
2. When Froude number is equal to one (Fe = 1), the flow is critical flow.
3. When Froude number is greater than one (Fe > 1), the flow is supercritical flow.
Q9. Define streamline.
Ans. A streamline is a hypothetical line within the flow where the tangent at any point on the line represents the velocity there.
Q10. What is stream tube ?
Ans. A fluid mass enclosed by a collection of streamlines is called a stream tube. Current filament refers to a stream tube’s contents.
Q11. What do you understand by circulation ?
Ans. The line integral of the tangential velocity about a closed path is what is known as circulation (contour). Integration can be used to obtain the circle’s path around regular curves.
Q12. Write down the definition of stream function ?
Ans. A stream function is a function of space and time that produces a velocity component that is at right angles to any direction when its partial derivative is applied to it. It is denoted by ψ
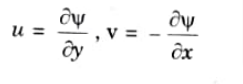
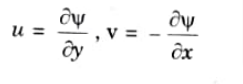
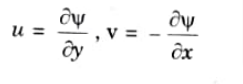
Q13. Define velocity potential function.
Ans. A scalar function of space and time called a “velocity potential function” is described as having a negative derivative with respect to each direction that corresponds to the fluid velocity in that direction.It is denoted by Φ.
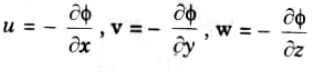
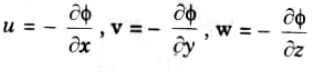
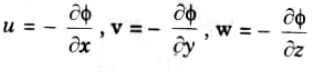
Q14. What do you understand by rate of flow ?
Ans. The amount of liquid that passes through a pipe or channel once every second is known as the rate of flow. It is expressed as,
Q = Av
Where, Q = Discharge.
A = Cross-sectional area of pipe.
v = Velocity.
Q15. The velocity distribution between two parallel plate is given by u = (a2 – y2), where u is the velocity at a distance y from the middle of the two plates. Find the expression for stream function.
Ans. Given : u = (a2 – y2)
To Find : Expression for stream function.
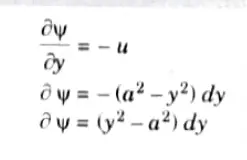
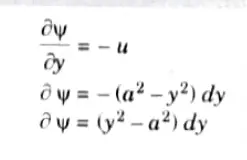
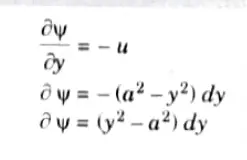
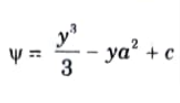
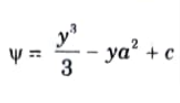
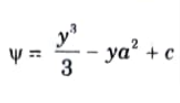
Q16. Find the frequency of oscillation when a 72 km/hr wind blows across a telephone wire of 3 mm diameter. Take v = 1.5 x 10-5 m2/s.
Ans. Given : v= 72 km/hr 20 m/sec, d=3 mm 3x 10-3
Alt= 1.5x 10-5 m/s
To Find: Frequency of oscillation.
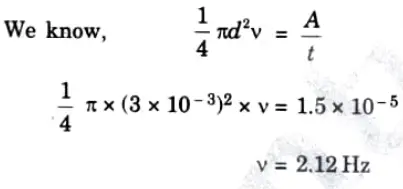
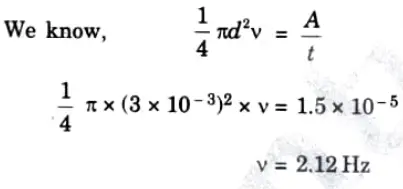
Unit – 3 (Potential Flow, Bernoulli’s Equation and its Application)
Q1. Define source flow.
Ans. The flow that originates at a place and spreads out uniformly in all directions along a plane is known as the source flow.
Q2. Explain sink flow.
Ans. The sink flow is the flow in which fluid travels continuously and radially inwards toward its point of disappearance. The source flow is exactly opposite of this flow.
Q3. What is doublet?
Ans. When a source and sink pair approach one another in such a way that their distance (2a) approaches zero and their product (2a.q), where q is the discharge, remains constant, this is known as a doublet.
Q4. State the assumptions of Bernoulli’s equation.
Ans. Assumptions are as follows:
- 1. Fluid ideal i.e., viscosity is zero.
- 2. Flow is steady.
- 3. Flow is incompressible.
- 4. Flow is irrotational.
Q5. What is venturimeter ?
Ans. A venturimeter is a tool used to gauge how quickly a fluid is moving through a pipe.
Q6. Diseuss orifice meter.
Ans. An orifice metre is a tool for calculating the flow rate of a fluid via a pipe. In comparison to a venturimeter, it is a less expensive gadget.
Q7. Define Pitot tube.
Ans. A pitot tube is a tool used to gauge the flow rate in any point or channel.
Q8. What is stagnation point ?
Ans. The point at which the fluid’s velocity zeros out is known as the stagnation point.
Q9. Differentiate between notch and weir.
Ans.
| S. No. | Notch | Weir |
| 1. | It is a tool used to gauge how quickly a liquid is moving through a tank or a narrow channel. | A weir is a building made of masonry or concrete that is positioned in an open channel where the flow occurs. |
Q10. Write the advantages of triangular notch or weir over rectangular notch or weir.
Ans. Following are the advantages
1. Ventilation of a triangular notch is not necessary.
2. It is best to use a triangular notch or weir when measuring low discharge.
3. For the computation of discharge, etc., just one reading (H) is necessary in the case of a triangular notch.
Q11. Write down minor energy losses in pipes.
Ans. The minor energy losses in pipes due to following ways:
1. Sudden expansion of pipe 2. Sudden contraction of pipe
3. Bend in pipe 4. Pipe fitting
5. An obstruction in pipe
Q12. What do you understand by TEL and HGL ?
Ans. TEL: The definition of a total energy line (TEL) is a line that expresses the sum of the pressure head, datum head, and kinetic head of a flowing fluid in a pipe with respect to a reference line.
HGL: Hydraulic gradient line is defined as the line which gives the sum of pressure head
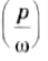
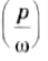
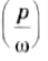
and datum head (z) of a flowing fluid with respect to some reference line.
Q13. What is siphon ?
Ans. When two reservoirs are separated by a hill or high level ground, a siphon—a long, curved pipe is used to move liquid from one reservoir at a higher elevation to another reservoir at a lower level.
Q14. Define water hammer in pipes.
Ans. The pipe walls get a pounding effect from the high pressure wave. In pipelines, this occurrence is referred to as water hammer.
Q15. On what factors, does water hammer pressure depend?
Ans. Water hammer pressure depends upon the following factor :
1. Velocity of flow,
2. Length of pipe,
3. Time taken to close the valve, and
4. Elastic properties of the material of pipe.
Q16. Define surface loss.
Ans. Surface loss is the loss of pressure or head in a pipe flow caused by the viscosity of the fluid close to the pipe’s surface.
Q17. Write practical application of Bernoulli’s equation.
Ans. All issues involving incompressible fluid flow and energy considerations are addressed by Bernoulli’s equation. But we shall consider its application to the following measuring devices
i. Venturimeter.
ii. Orifice meter
iii. Pitot-tube.
Q18. Discuss about Euler’s equation of motion.
Ans. The forces resulting from gravity and pressure are taken into consideration in Euler’s equation of motion. This is created by timing a fluid element’s motion along a streamline.
Euler’s equation is given by.
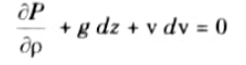
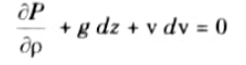
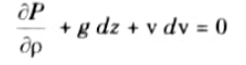
Unit – 4 (Flow Through Pipes)
Q1. Define Stoke’s law.
Ans. According to Stoke’s law, a drag force (F) acting on the sphere moving with a constant velocity in a viscous fluid of viscosity (μ)is given by,
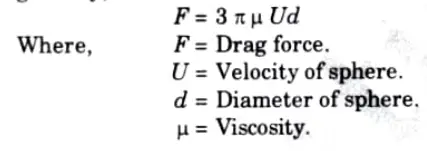
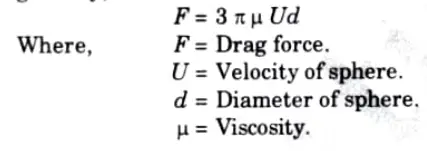
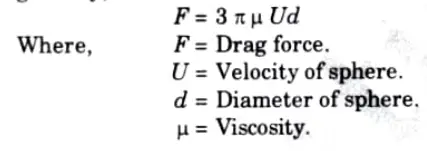
Q2. What do you understand by kinetic energy correction factor ?
Ans. The ratio of the kinetic energy of the flow per second based on real velocity across a segment to the kinetic energy of the flow per second based on average velocity across the same section is known as the kinetic energy correction factor.
Q3. When will a laminar flow change to turbulent flow ?
Ans. A laminar flow may change to turbulent flow when
1. There is an increased velocity of flow.
2. There is an increased diameter of pipe.
3. The viscosity of fluid is decreased.
Q4. Give the classification of turbulent flow.
Ans. The turbulent flow can be classified as follows:
1. Wall turbulence,
2. Free turbulence, and
3. Convective turbulence.
Q5. Define isotropic turbulence.
Ans. Isotropic turbulence is defined as turbulence whose characteristics, particularly statistical correlations, do not depend on direction.
Q6. Define homogeneous turbulence.
Ans. Homogeneous turbulence is defined as having a quantitative structure in all areas of the flow fluid.
Q7. Explain kinematic eddy viscosity.
Ans. The ratio of (eddy viscosity) and p (mass density) is known as kinematic eddy viscosity and is denoted by ε. Mathematically,
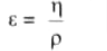
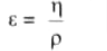
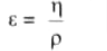
Q8. Define boundary layer thickness.
Ans. The distance from the solid body’s boundary, measured in the Y direction, to the point where the fluid’s velocity is roughly equal to 0.99 times that of the free stream of the fluid is known as the boundary layer thickness.
Q9. Define the displacement thickness?
Ans. The distance that the solid body’s boundary should be shifted by, measured perpendicular to the boundary, in order to make up for the flow reduction caused by the creation of boundary layers is known as the displacement thickness.
Q10. Explain momentum thickness.
Ans. Momentum thickness is the distance, measured perpendicular to the solid body’s border, by which the boundary should be moved in order to make up for the loss of fluid momentum caused by the creation of a boundary layer.
Q11. What is energy thickness?
Ans. Energy thickness is the distance, measured perpendicular to the solid body’s border, by which the boundary should be moved in order to make up for the loss of fluid kinetic energy caused by the creation of a boundary layer.
Q12. Define turbulent boundary layer and laminar sub layer.
Ans. Turbulent boundary: The boundary layer is turbulent and thickens further downstream toward the transition zone. Turbulent boundary layer is the name given to this boundary layer. Laminar sublayer: This is the area of the plate’s solid surface that is adjacent to the boundary layer zone.
Q13. Enumerate the methods of preventing the separation of boundary layer.
Ans. Following are the methods for preventing the separation of boundary layer:
1. Suction of the slow moving fluid by a suction slot.
2. Supplying additional energy from a blower.
3. Providing guide blades in a bend.
4. Acceleration of fluid in the boundary layer.
5. Using stream lined body shape.
Q14. Explain impulse momentum equation.
Ans. It states that the impulse of force F acting on a fluid mass m in a short interval of time dt is equal to the change of momentum d(mv) in direction of force.
Mathematically,
Fdt = d(mv)
Q15. What do you understand by shape factor ?
Ans. The ratio of momentum thickness (Ө to displacement thickness (δ)*) is known as shape factor.
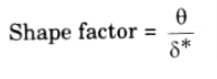
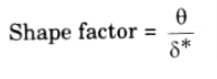
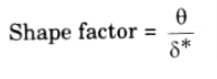
Q16. What is flow separation ?
Ans. The separation of a boundary layer from a surface and into a wake is known as flow separation or boundary layer separation. After passing the thickest portion of the stream line body or going through a widening passage, separation occurs in flow that is slowing down with pressure increasing.
Unit – 5 (Drag and Lift)
Q1. Explain the drag and lift.
Ans. a. Drag: The component of the total force (FR) in the direction of motion is called drag. This component is denoted by Fp.
b. Lift : The component of the total force (Fp) in the direction perpendicular to the direction of motion is known as lift. This is denoted by FL.
Q2. What is average coefficient of drag?
Ans. Average coefficient of drag is defined as the ratio of the total drag
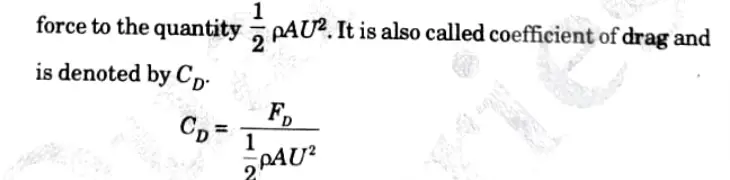
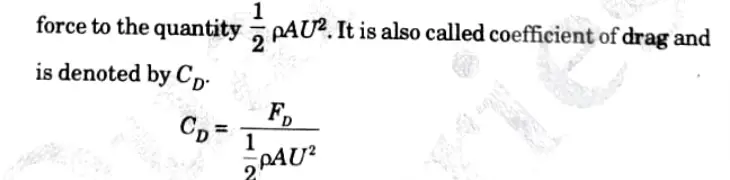
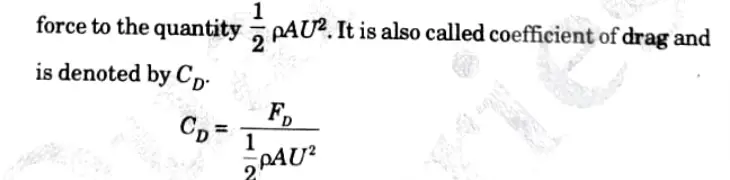
Q3. What are the Magnus effects ?
Ans. A lift force is generated on a cylinder when it is spun in a uniform flow. The Magnus effect is the name for this phenomena.
Q4. Distinguish between model and prototype.
Ans.
| S. No. | Model | Prototype |
| 1. | It is not necessarily functional. | It is fully functional. |
| 2. | Used for visual demonstration of product. | Used for performance evaluation and further improvement of product. |
Q5. What is the meaning of geometric similarity between model and prototype ?
Ans. If all of the related linear dimensions in the model and prototype have the same ratio, there is said to be geometric resemblance between them.
Q6. Discuss kinematic similarity.
Ans. Kinematic similarity refers to how motion in a model and prototype is similar. Therefore, if the ratios of the velocity and acceleration at the respective sites in the model and the prototype are the same, a relationship is said to exist between them.
Q7. What is dynamic similarity ?
Ans. The similarity of forces is known as dynamic similarity. If identical sorts of forces are parallel and carry the same ratio at all of the relevant sites at both the prototype and the model, the flows will be dynamically equivalent.
Q8. Enumerate the different model laws or similarity laws.
Ans. Following are the model laws:
1. Reynolds model law,
2. Froude model law,
3. Euler model law,
4. Weber model law, and
5. Mach model law.
Q9. Explain undistorted and distorted models.
Ans. a. Undistorted Models: Models that are geometrically similar to their prototypes are said to be undistorted models. In other words, a model is said to be undistorted if the scale ratio for its linear dimensions is the same as that of its prototype.
b. Distorted Models: If a model differs geometrically from its prototype, it is said to be distorted. Different scale ratios are used for the linear dimensions of this type of model.
Q10. State the Buckingham’s π-theorem.
Ans. According to the theorem, if a physical phenomena has n variables (independent and dependent variables) and m basic dimensions (M, L, and T), then the variables can be grouped into (n – m) dimensionless terms.
Q11. Where is Mach model law applicable ?
Ans. The Mach model law finds application in the following:
1. Aerodynamic testing,
2. Phenomena involving velocities exceeding the speed of sound,
3. Hydraulic model testing for the cases of unsteady flow, especially water hammer problems, and
4. Underwater testing of torpedoes.
Q12. What are the uses of Weber model law?
Ans. Weber model law is applied in the following flow situations
1. Flow over weirs involving very low heads,
2. Very thin sheet of liquid flowing over a surface,
3. Capillary waves in channels,
4. Capillary rise in narrow passages,
5. Capillary movement of water in soil.
Q13. Write down the application of Reynolds model law?
Ans. 1. Motion of aeroplanes,
2. Flow of incompressible fluid in closed pipes,
3. Motion of submarines completely under water, and
4. Flow around structures and other bodies immersed completely under moving fluids.
Q14. What is the necessity of Froude model law ?
Ans. 1. Free surface flows such as flow over spillways, sluices etc.,
2. Flow of jet from an orifice or nozzle,
3. Where waves are likely to be formed on the surface, and
4. Where fluids of different mass densities flow over one another.
Q15. What do you mean by “Dimensional Analysis”?
Ans. It is a mathematical method for resolving various engineering issues that makes use of the study of dimensions.
Q16. What do you understand by dimensional homogeneity ?
Ans. Dimensional homogeneity refers to an equation’s terms having the same dimensions on both sides.
Q17. Explain bluff and streamlined body.
Ans. Streamlined Body : A “streamlined body” is a body whose surface, when positioned in a flow, coincides with the streamlines.Bluff Body : A “bluff body” is a body whose surface does not match the streamlines when it is positioned in a flow.



Important Question with solutions | AKTU Quantums | Syllabus | Short Questions
Fluid Mechanics Btech Quantum PDF, Syllabus, Important Questions
| Label | Link |
|---|---|
| Subject Syllabus | Syllabus |
| Short Questions | Short-question |
| Important Unit-1 | Unit-1 |
| Important Unit-2 | Unit-2 |
| Important Unit-3 | Unit-3 |
| Important Unit-4 | Unit-4 |
| Important Unit-5 | Unit-5 |
| Question paper – 2021-22 | 2021-22 |
Fluid Mechanics Quantum PDF | AKTU Quantum PDF:
| Quantum Series | Links |
| Quantum -2022-23 | 2022-23 |
AKTU Important Links | Btech Syllabus
| Link Name | Links |
|---|---|
| Btech AKTU Circulars | Links |
| Btech AKTU Syllabus | Links |
| Btech AKTU Student Dashboard | Student Dashboard |
| AKTU RESULT (One VIew) | Student Result |
