All Short Important Questions that Might Be Asked During Future Network Analysis and Synthesis Exams Are Provided. I sincerely hope you would appreciate it and continue to follow Bachelorexam.com.
UNIT:-01 (Node and Mesh Analysis)
1. Write Kirchhoff’s law.
Ans. Kirchhoff’s current law (KCL):At all times, the algebraic total of the branch currents at a node equals zero.
Σ i = 0
Kirchhoff’s voltage law (KVL) : All branch voltages around every closed loop in a network have an algebraic total that is always equal to zero.
Σ V = 0
2. What is duality and write any two limitations of dual network ?
Ans. Currents and voltages are switched during a duality transition. If two phenomena can be described by equations with the same mathematical form, they are said to be dual.
Limitations:
- 1. Due of its non-linearity, power has no dual network.
- 2. A circuit element might not have a dual network like mutual inductance even though linearity is applicable.
3. Define ideal voltage and current source.
Ans. Ideal voltage source : If the internal resistance of a voltage source is zero, then it is an ideal source element capable of supplying any current at a constant voltage. The terminal voltage is unaffected by the quantity of load current since it is equal to the voltage across the source.
Ideal current source: Ideal current source is a source that delivers a constant current to a load regardless when the load’s impedance changes. Regardless of the load impedance, the current delivered by such a source should be constant.
4. What information is obtained from the loop?
Ans From a loop, the algebraic sum of potential differences is obtained.
5. Define nodes.
Ans. A link between two or more circuit components is referred to as a node.
6. Explain mesh in electrical network.
Ans A closed path surrounding a circuit that doesn’t have any other closed paths inside of it is referred to as a mesh.
7. What are the utilities of duality of network?
Ans It makes resolving challenging network issues relatively simple.
8. What is a loop ?
Ans A loop is a closed path that is created by starting at a node and following the path back to it without crossing any intermediary nodes more than once.
9. When is mesh analysis preferred over nodal analysis ?
Ans Mesh analysis is preferred over nodal analysis :
- 1. when there are several voltage sources or simply voltage sources in the circuit.
- 2. when there are more meshes than nodes in the circuit.
- 3. when it is necessary to determine many currents.
10. When is nodal analysis preferred over mesh analysis ?
Ans Nodal analysis is preferred over mesh analysis:
- 1. When there are one or more current sources present in the circuit.
- 2. When there are fewer nodes in the circuit than meshes.
- 3. When the need for node voltage arises.
- 4. The only method available when the network is non-planar is nodal analysis.
11. If a circuit has B branches and N nodes including reference nodes, how many meshes are there?
Ans. Number of meshes = Branches – (Nodes -1),
M = B – (N – 1)
12. What is a planar circuit ?
Ans A circuit that may be drawn on a level surface without crossings is called a planar circuit.
13. What is a non-planar circuit ?
Ans A circuit that cannot be drawn without crossings on a flat surface is said to be non-planar.
14. When are two networks said to be duals of each other ?
Ans If two networks are controlled by the same set of equations, they are said to be duals of one another.
15. Write pairs of dual terms for electrical networks.
Ans. Current-voltage, resistance-conductance, inductance-capacitance, loop-node, series-parallel, open-short, and KCL-KVL are a few dual terminology for electrical circuits.
UNIT:-02 (Network Theorems)
1. State superposition theorem.
Ans Any element of a linear bilateral RLC network with multiple independent voltage and current sources would respond according to the algebraic sum of the responses that each source working independently would provide.
2. Write the application of superposition theorem with its statement.
Ans
- 1. This proposition holds true for every kind of linear circuit with time-varying or time-invariant components.
- 2. When the circuit contains a lot of different independent sources, this theorem is utilised to determine the current or voltage in a branch.
3. Give limitations of the superposition theorem.
Ans
- 1. Not suitable to networks that have unilateral or non-linear components.
- 2. Does not apply to nonlinear characteristics like power.
- 3. Power relations are not covered by this theorem.
4. Write the statement of Thevenin’s theorem.
Ans An equivalent circuit made up of a voltage source can substitute any two-terminal linear network with sources (generators) and impedances. The open circuit voltage between the network terminals is Vth in series with the impedance, and Zth is the impedance measured between the terminals of the network after all energy sources have been removed.
5. What are the limitations of Thevenin’s theorem ?
Ans
- 1. Loads that are magnetically connected to other branches of the circuit are not covered by this theorem.
- 2. Unilateral and non-linear networks cannot be used to prove this theorem.
- 3. Active load is excluded from this theorem.
- 4. There should be no dependent sources in the load.
6. State the reciprocity theorem and write its advantages.
Ans. It claims that regardless of the position of excitation and response inside a linear time-varying network, the reaction to excitation remains constant.
Advantages:
- 1. Applicable to a network made up of transformers and linear, time-invariant, bilateral passive devices.
- 2. In order to apply this theorem, all initial conditions must be zero and we must only take into account the zero-state response.
7. Mention the important points of Norton’s theorem.
Ans
- 1. Applicable to any linear, bilateral, active network.
- 2. Inapplicable to non-linear and unilateral networks.
- 3. This theorem is inapplicable for active load.
8. What do you understand about the maximum power transfer theorem?
Ans When the impedance of one network is the complex conjugate of the other, the maximum power can be absorbed by another network connected to it at two terminals.
9. What do you understand about the compensation theorem?
Ans Any branch carrying a current I in a linear, bilateral, active network would experience changes in impedance Z that are the same as those that would have been brought about by the injection of a voltage source of -16Z in the modified branch.
10. State Tellegen’s theorem.
Ans. Tellegen’s theorem states that for a network of n elements and e nodes, ifa set of current passing through various elements be i1, i2, …., ie satisfying KCL and its set of voltages be V1, V2, …., Ve satisfying KVL for every loop, then Tellegen’s theorem is
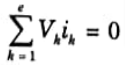
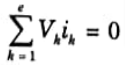
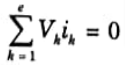
11. What is the condition for maximum power transfer in a network? Also mention any two applications of maximum power transfer theorem.
Ans Condition for maximum power transfer : Maximum power will be transferred when the load resistance is equal to the source resistance i.e.,
Rs = RL
Applications of maximum power transfer theorem:
1. In the communication system.
2. In car engines.
12. Where is the compensation theorem mainly used ?
Ans Bridge and potentiometer circuits.
13. What is the limitation of the reciprocity theorem?
Ans It does not apply to multisource networks and only applies to single source networks. Additionally, neither time-varying elements nor dependent sources should be present in the network.
14. Why does the superposition principle cannot be applied directly to find power?
Ans. Due to the non-linear relationship between current and power, the superposition principle cannot be used to directly determine power.
15. If a circuit contains no independent energy sources, what are the values of VTh and IN
Ans. Both VTH and yN are zero, and the circuit has only the equivalent Thevenin resistance.
16. What is the efficiency of the circuit under the condition of maximum power transfer ?
Ans. When power transfer is at its highest, the circuit’s efficiency is 50%.
UNIT:-03 (Fourier Series)
1. What is the Fourier series?
Ans The Fourier series is a linear combination of orthogonal functions that describes signals over a given period of time.
2. Write Dirichlet’s conditions for the existence of Fourier series.
Ans. Dirichlet’s conditions are the circumstances in which a periodic signal can be represented by a Fourier series. They areas follows:
In each period,
i The function x(t) must be a single valued function.
ii. The function x(t) has only a finite number of maxima and minima.
iii. The function x(t) has a finite number of discontinuities.
iv. The function x(t) is absolutely integrable over one period, that is
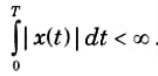
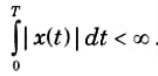
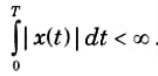
3. Discuss even symmetry and odd symmetry.
Ans. A function x(t) is said to have even symmetry, if x(t) = x(-t).
A function z(t) is said to have odd symmetry, if x(-t) = – x(t).
4. What is half wave symmetry ?
Ans A function x(t) is said to have half wave symmetry, if
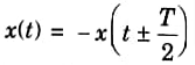
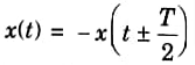
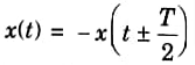
When it exists, only odd harmonics will be present.
5. What do you understand about the Fourier transform?
Ans. Using the Fourier transform, signals can be converted from the continuous time domain to the frequency domain and vice versa.
6. Mention the merits of Fourier transform.
Ans.
- 1. It can be used to retrieve the original time function in a singular way.
- 2. The Fourier transform can be used to assess convolution integrals.
7. Differentiate between Fourier series and Fourier transform.
Ans.
| S. No. | Fourier series | Fourier transform |
| 1. | The representation of a signal over a certain interval of time in terms of linear combination of orthogonal functions is called Fourier series | Fourier transform is a transformation technique which transforms signals from the continuous time domain to corresponding frequency domain and vice-versa. |
| 2. | It is only applicable for periodic signals. | It is applicable for both periodic and non-periodic signals |
| 3. | The spectrum is discrete in nature. | The spectrum is continuous in nature. |
8. Give the applications of Fourier transform.
Ans.
- 1. Analysis of LTI systems
- 2. Cryptography
- 3. Signal analysis
- 4. Signal processing
9. What is the frequency spectrum?
Ans The magnitude spectrum is the plot of |H(o)| versus a, and the phase spectrum is the plot of ZH(o) versus 0 1s. The frequency response is the sum of the amplitude spectrum and phase spectrum.
10. What are the cases of unbalanced load in a three-phase
system ?
Ans
- 1. Unbalanced delta-connected load
- 2. Unbalanced three-wire, star-connected load
- 3. Unbalanced four-wire, star-connected load
11. Find the Fourier transform of x(t-t0)
Ans. Let the function is x(t-t0)
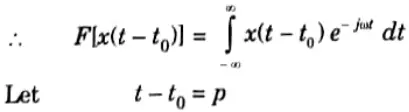
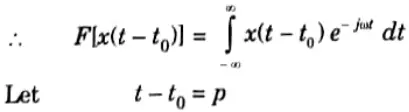
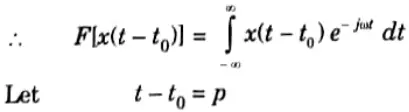
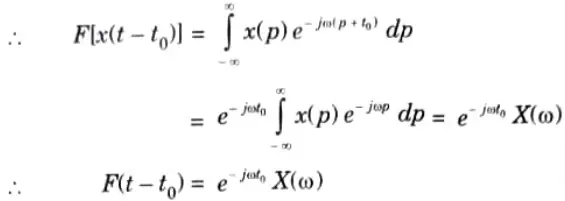
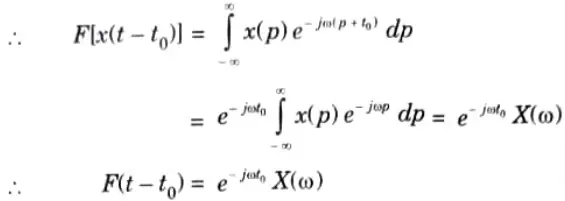
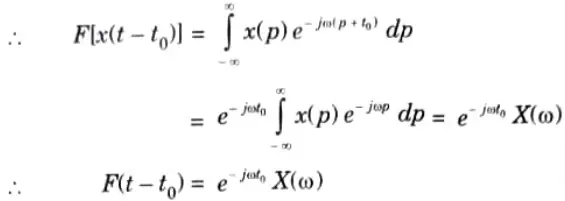
12. What are the methods to solve three-phase, three-wire, star connected unbalanced loads 2
Ans
1. Star-delta transformation
2. Millman’s method.
13. Which method is used to measure the power in balanced and unbalanced load ?
Ans The power in both balanced and unbalanced loads can be measured using the three-wattmeter method and the two-wattmeter method, but the power in just balanced loads can be measured using the one-wattmeter method.
14. How are powers in a three-phase balanced load calculated
Ans.
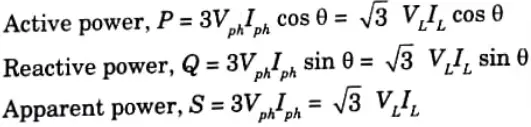
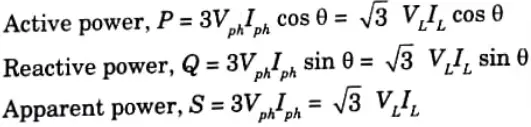
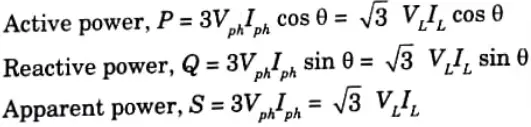
15. What is the relation between line and phase voltages in a star-connected system ?
Ans.
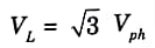
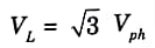
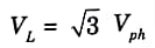
16. What is the relation between line and phase current in a delta-connected system ?
Ans.
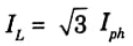
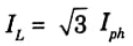
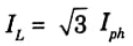
UNIT:-04 (Laplace Transform)
1. Give the advantages of Laplace transform.
Ans.
- 1. Laplace transforms converge signals that do not converge in Fourier transforms.
- 2. S-domain multiplication can be used to obtain convolution in the time domain.
- 3. System integro-differential equations can be transformed into straightforward algebraic equations. Laplace transforms can therefore be used to quickly investigate LTI systems.
2. Write the disadvantages of Laplace transform.
- Ans. 1. The Laplace domain integral representation is challenging.
- 2. The system’s frequency response cannot be approximated or drawn. Only the pole-zero layout can be drawn in its place.
- 3. S =jW is used only for sinusoidal steady-state analysis.
3. Compare Laplace transform and Fourier transform.
Ans.
| S. No. | Fourier transform | Laplace transform |
| 1. | It does not have any convergence factor. | It has a convergence factor. |
| 2. | It cannot be used to analyse unstable systems. | It can be used to analyse even unstable system. |
4. Mention the applications of Laplace transform.
Ans
- 1. For system modeling.
- 2. Used to solve differential equations.
- 3. Used in electrical circuits for the analysis of linear time invariant systems.
5. How are resistance (R), inductance (L) and capacitance (C) transformed into s-domain 1
Ans Remains as R in s-domain,
L transforms to Ls in s-domain,
C transforms to 1/ Cs in s-domain.
6. What are the basic elements in electrical circuits ?
Ans.
- 1. Resistor,
- 2. Inductor and
- 3. Capacitor.
7. Write the v-i relationship in the case of
i. Pure resistance,
ii. Pure inductance
iii. Pure capacitance.
Ans.
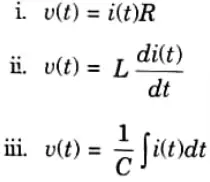
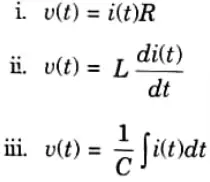
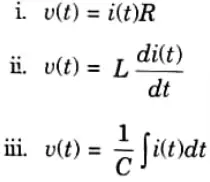
8. Define the transfer function of a system.
Ans When the beginning conditions are ignored, the transfer function of a system is defined as the ratio of the Laplace transform of the output to the Laplace transform of the input.
9. State initial value theorem.
Ans.
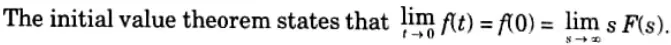
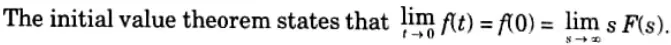
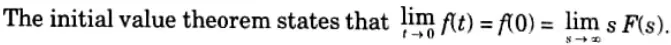
10. State final value theorem.
Ans.
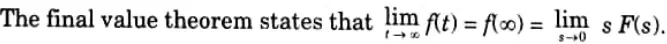
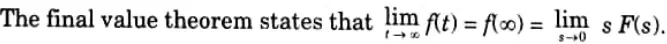
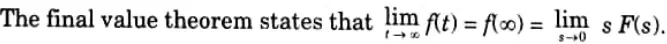
11. When does the Laplace transform of a function f(t) exist ?
Ans.



12. What is the relation between Laplace transform and Fourier transform ?
Ans.



UNIT:-05 (Transient Behavior)
1. Write system stability conditions.
Ans. 1. All of a linear system’s poles must have negative real parts, or they must be located on the left side of the s-plane, for the system to be stable.
2. A system is considered marginally stable if one or more of its poles lie on the hypothetical axis of the s-plane and its natural response contains non-decaying oscillation components.
2. What are the advantages of the transfer function ?
Ans.
- 1. It provides a straightforward algebraic equation.
- 2. It directly provides the system’s poles and zeros.
- 3. It is simple to assess the system’s stability.
- 4. It is simple to calculate the system’s output for any input.
3. What are the disadvantages of transfer function ?
Ans
- 1. It works with the LTI system.
- 2. It does not consider the beginning situation.
- 3. Only applicable for a single input and output.
- 4. It is impossible to determine controllability and observability.
4. What are poles and zeros ?
Ans Crucial complex frequencies known as poles and zeros are critical complex frequencies when the network function has infinite value and zero value, respectively.
5. What do complex frequencies corresponding to system poles and excitation poles reveal ?
Ans: While complex frequencies corresponding to excitation poles disclose the forced or steady reaction and depend on the driving force, complex frequencies corresponding to system poles reveal the system’s natural or transient response and depend on the system function.
6. For a two-port network define the driving point functions and transfer functions
Ans Driving point functions:
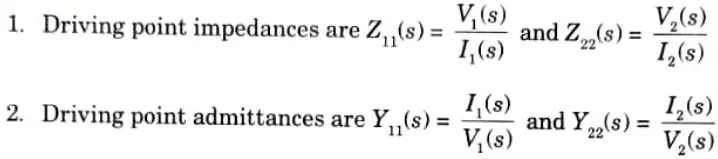
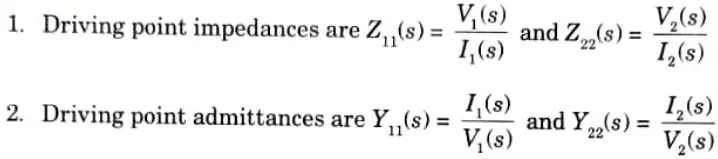
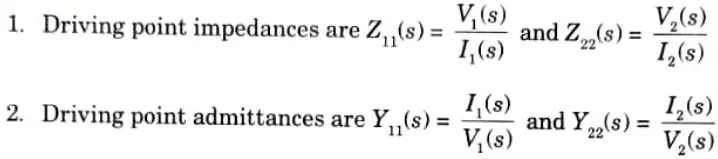
Transfer functions:
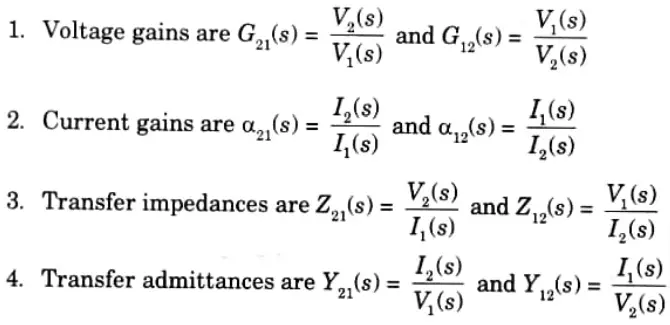
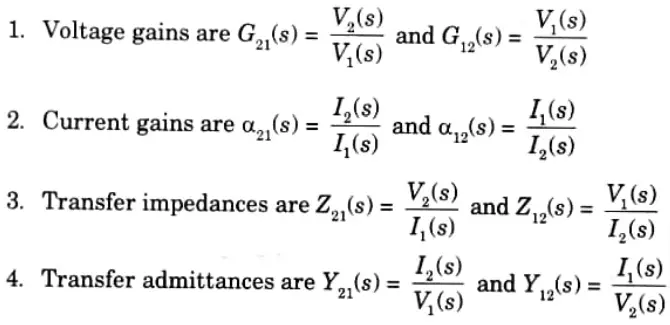
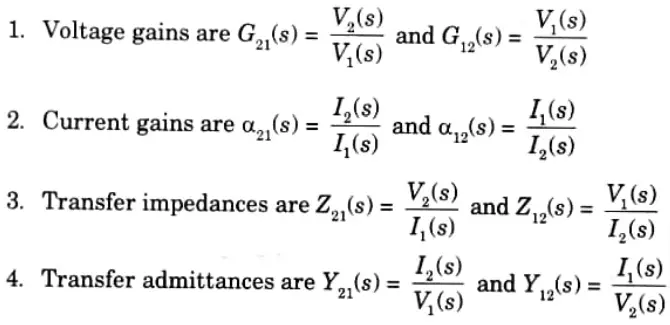
7. What is a filter ?
Ans A filter is a frequency selective network that completely suppresses all other bands while freely passing the chosen bands of frequencies.
8. How are filters classified ?
Ans The four common types of filters are low-pass, high-pass, band-pass, and band-elimination.
9. What is a low-pass filter?
Ans A low-pass filter is one that attenuates all frequencies above the cut-off frequency f while permitting all frequencies below it to pass without attenuation.
10. What is a high-pass filter ?
Ans A high-pass filter attenuates all frequencies below a specified cut-off frequency f while allowing all frequencies above that frequency to pass.
11. What is a band-pass filter ?
Ans A band-pass filter is one that attenuates all frequencies above and below two predetermined cut-off frequencies while passing all frequencies in between.
12. What is a band-stop filter ?
Ans. A band-stop filter is one that attenuates all frequencies between two preset frequencies while passing all frequencies outside of the range.
13. Why are z-parameters called open circuit impedance parameters ?
Ans. Z-parameters are referred to as open since they are measured in terms of impedance and are determined in an open circuit.
14. Why ABCD-parameters are called transmission parameters ?
Ans Due to its widespread application in transmission line theory and cascade networks, ABCD-parameters are also known as transmission parameters.
15. Where are hybrid parameters mostly used?
Ans. Transistor circuits frequently use hybrid parameters.
16. What is the transmission matrix of a cascade of two-part networks?
Ans The sum of the transmission matrices of each individual two-port network makes up the transmission matrix of a cascade of two-port networks.



Syllabus | Quantums | notes | Important Questions
Network Analysis and Synthesis Important Links:
| Label | Link |
|---|---|
| Subject Syllabus | Syllabus |
| Short Questions | Short-question |
| Important Unit-1 | Unit-1 |
| Important Unit-2 | Unit-2 |
| Important Unit-3 | Unit-3 |
| Important Unit-4 | Unit-4 |
| Important Unit-5 | Unit-5 |
| Question paper – 2021-22 | 2021-22 |
Network Analysis and Synthesis Quantum PDF: | AKTU Quantum PDF:
| Quantum Series | Links |
| Quantum -2022-23 | 2022-23 |
AKTU Important Links | Btech Syllabus
| Link Name | Links |
|---|---|
| Btech AKTU Circulars | Links |
| Btech AKTU Syllabus | Links |
| Btech AKTU Student Dashboard | Student Dashboard |
| AKTU RESULT (One VIew) | Student Result |

3 thoughts on “Network Analysis and Synthesis Short Questions with solution.”